Project Outsourcing
Outsource your project to the MR CFD simulation engineering team. Our experts are ready to carry out every CFD project in all related engineering fields. Our services include industrial and academic purposes, considering the ANSYS Fluent software's wide range of CFD simulations. By outsourcing your project, you can benefit from MR CFD's primary services, including Consultation, Training, and CFD Simulation. The project freelancing procedure is as follows:
An official contract will be set based on your project description and details.
As we start your project, you will have access to our Portal to track its progress.
You will receive the project's resource files after you confirm the final report.
Finally, you will receive a comprehensive training video and technical support.
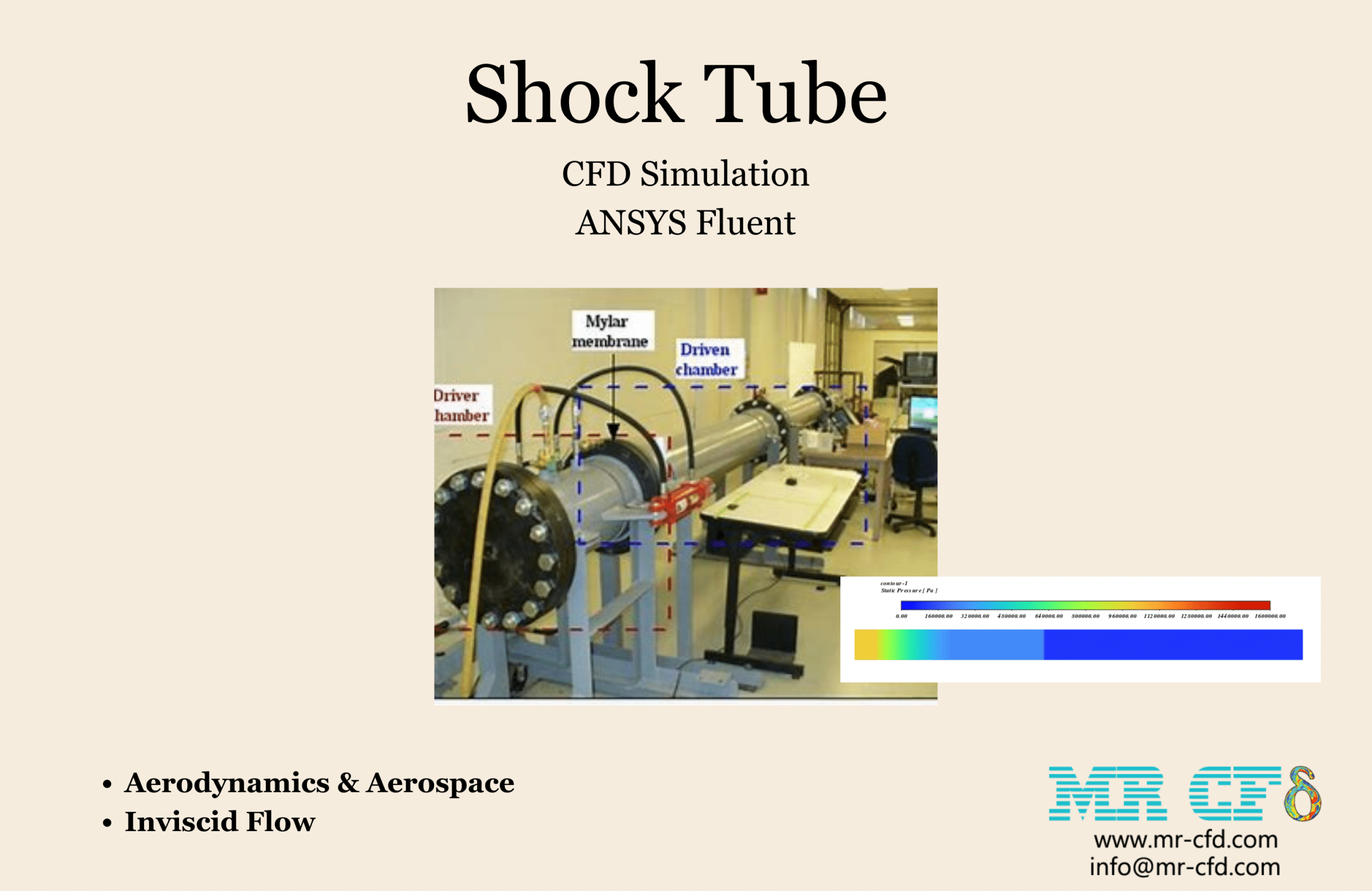
What is Inviscid Flow?
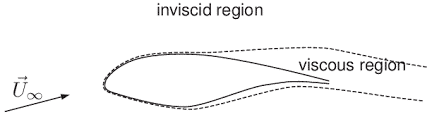
The inviscid-flow assumption means physically that viscous-shear and normal stresses are negligible. Thus, all of the viscous shear-stress terms on the force side of the momentum equations drop out, and the normal stresses due to viscosity. As a result, the only stresses acting on the body surface are the normal stresses due to pressure. While for viscous fluids, the viscous-shear stresses are assumed to be proportional to the rate of strain of a fluid particle, with the constant of proportionality as the viscosity coefficient. Thus, an assumption equivalent to that of negligible viscous stresses is that the viscosity coefficient is essentially zero.
Such a flow is termed inviscid (i.e., of zero viscosity). In effect, the boundary layer on the body’s surface is deleted by this assumption. This implies that the boundary layer must be very thin compared to a dimension of the body. The presence or absence of the boundary layer has a negligible effect relative to modifications to the body geometry as “seen” by the flow. The inviscid, incompressible-fluid model is often termed a perfect fluid (not to be confused with an excellent or ideal gas). The boundary layer in many practical situations is extremely thin compared to a typical dimension of the body under study. The body shape that a viscous flow “sees” is essentially the geometric shape. The exception is where the flow separates, and the boundary layer leaves the body, resulting in a significant change in the effective geometry of the body. Such separated regions occur on wings, for example, at large angles of attack.
However, the wing angle of attack of a vehicle at a cruise condition is only a few degrees, so the effects of separation are minimal. Thus, the inviscid-flow assumption provides useful results that closely match the experiment for conditions corresponding to cruise. The inviscid-flow model breaks down when large regions of separated flow occur. Because the presence of the boundary layer is neglected in perfect-fluid theory, the theory does not predict the frictional drag of a body; that must be left to viscous-flow theory. However, predictions for low-speed pressure distribution, lift, and pitching moment are valid within the framework of incompressible inviscid flow.
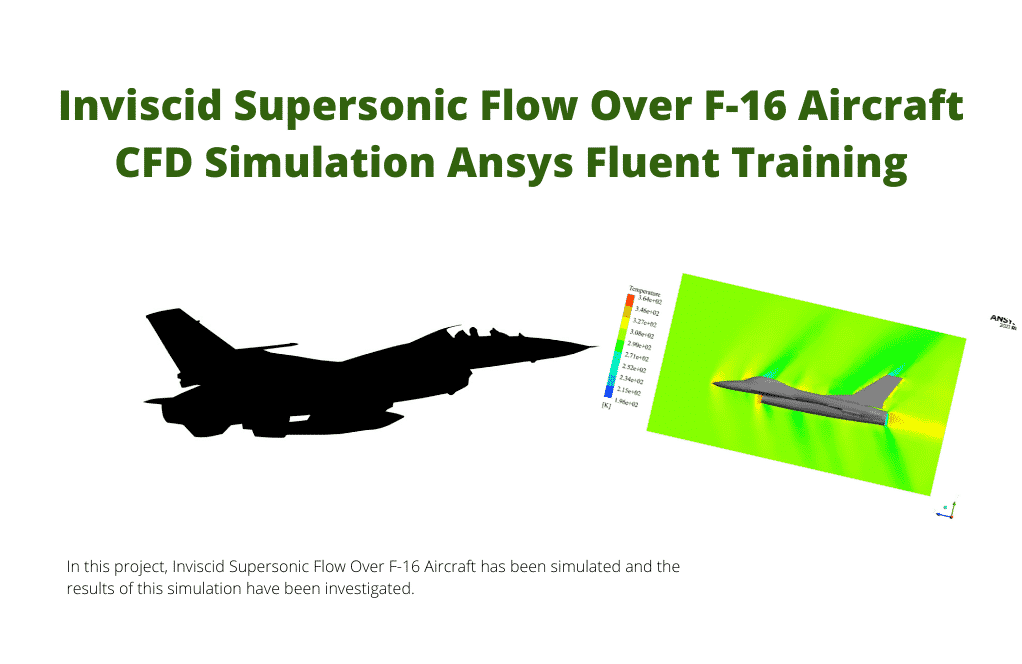
You can see the project Inviscid Supersonic Flow over F-16 Aircraft link below, which is carried out by the MR-CFD team:
MR-CFD, an Expert in the Field of CFD Simulations
With several years of experience in simulating various problems in various CFD fields using ANSYS Fluent software, the MR-CFD team is ready to offer extensive modeling, meshing, and simulation services based on the need of our customers. Our services are not limited to the mentioned subject, and the MR-CFD team is ready to undertake different and challenging projects. You can consult with our experts freely and without charge at first, and then order your project by sending the problem details to us using the following address.
By outsourcing your project to the MR-CFD as a CFD simulation freelancer, you will not only receive the related project’s files (Geometry, Mesh, …), but also you will be provided with an extensive tutorial video demonstrating how you can create the geometry, mesh, and define the needed settings(pre-processing, processing and post-processing) in the ANSYS Fluent software all by yourself. Additionally, post-technical support is available to clarify issues and ambiguities.