Fluidized Bed Bio-Reactor ANSYS Fluent Training
$160.00 Student Discount
- The problem numerically simulates the fluidized bed bio-reactor using ANSYS Fluent software.
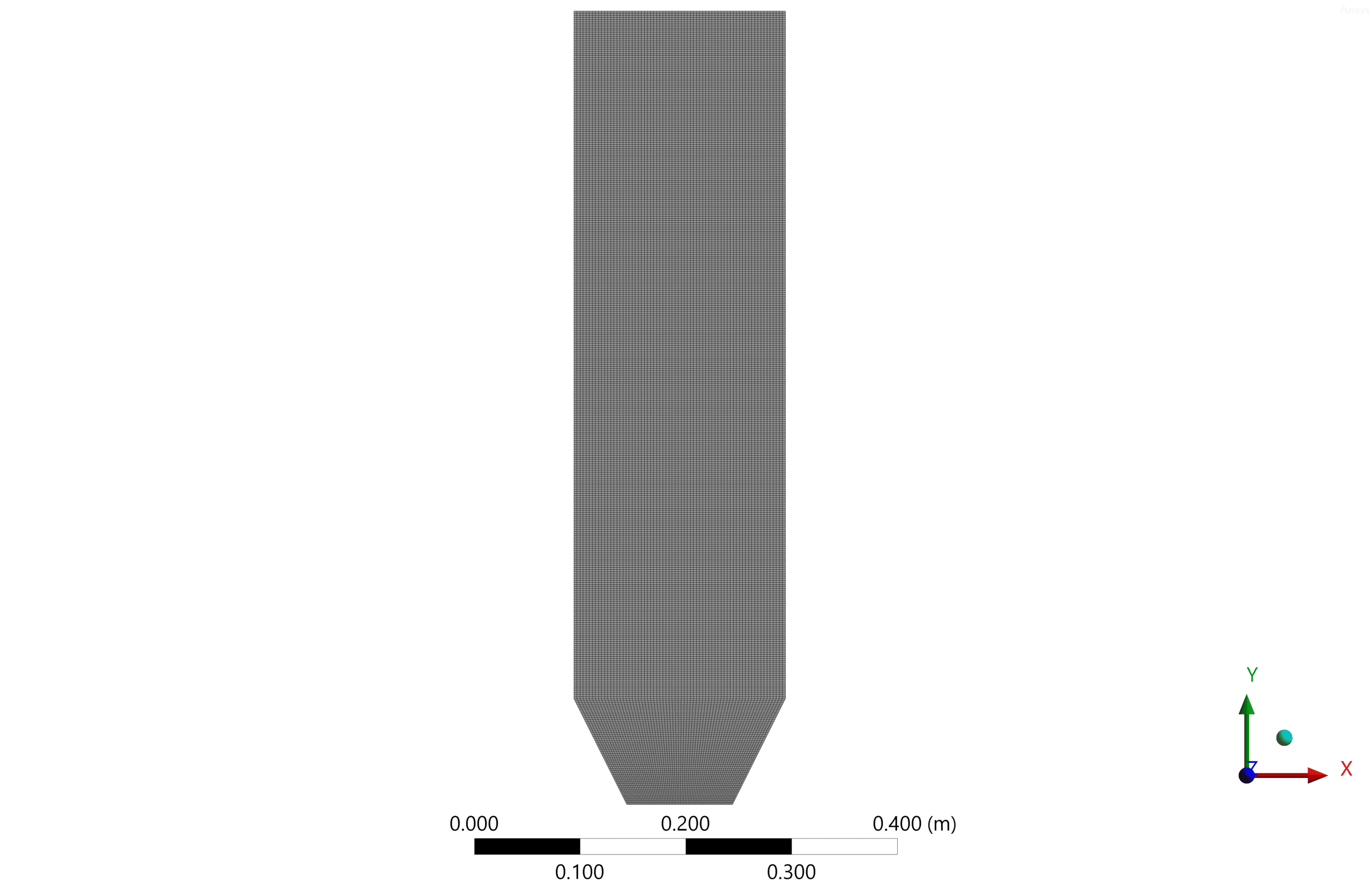
- The geometry is designed in a 2D model with the ANSYS Design Modeler software.
- We performed the structured mesh of the model with ANSYS Meshing software, and the element number equals 26,289.
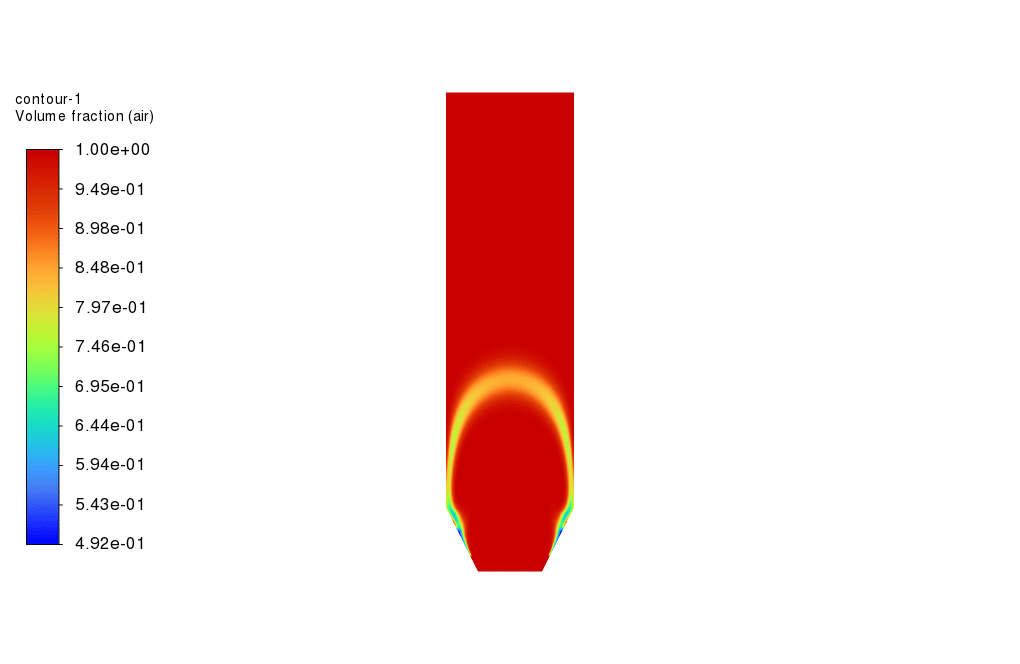
- The Eulerian model was used to simulate the multiphase behavior of the solution.
- The project is solved in transient state.
To Order Your Project or benefit from a CFD consultation, contact our experts via email ([email protected]), online support tab, or WhatsApp at +44 7443 197273.
There are some Free Products to check our service quality.
If you want the training video in another language instead of English, ask it via [email protected] after you buy the product.
Description
Description
This project investigates the fluidized bed bio-reactor. A fluidized bed bioreactor (FBR) is a type of device that can perform a variety of multiphase chemical reactions. In these types of reactors, a fluid (gas or liquid) is passed through a solid granular material (usually a catalyst) at a rate sufficient to suspend the solid and cause it to behave like a fluid.
This product is the third chapter of the Eulerian Multiphase Model Training Course.
This process, known as fluidization, gives FBR many important advantages. As a result, FBRs are used for many industrial applications such as biomedical and food industry.
The geometry of the present project is designed in Design modeler and meshed in ANSYS Meshing software. The mesh type is structured and the element number is equal to 26,289.
Fluidized Bed Bio-Reactor Methodology
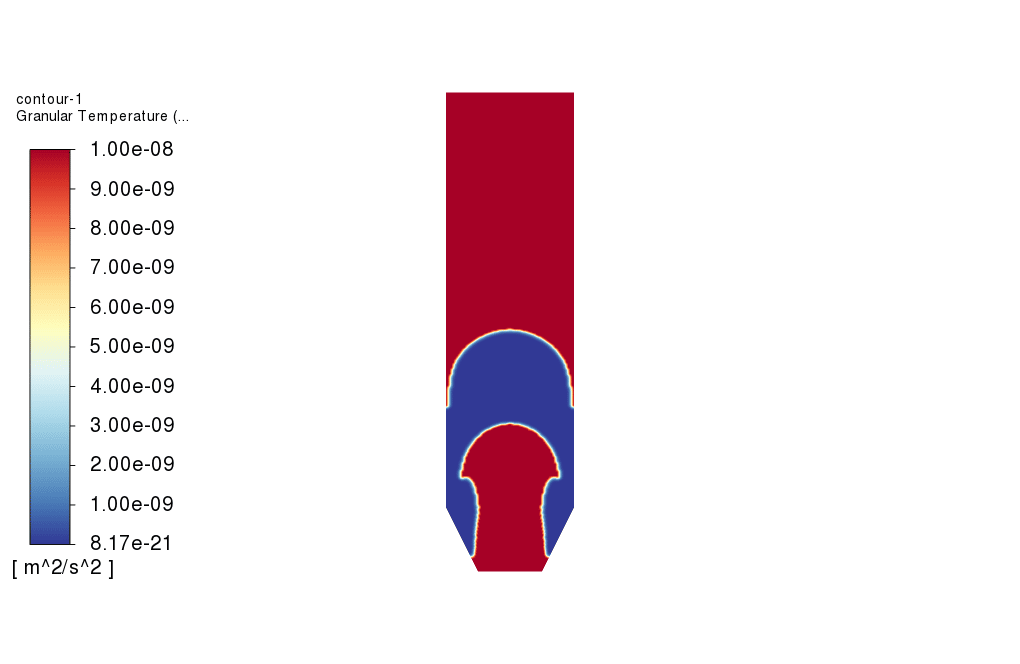
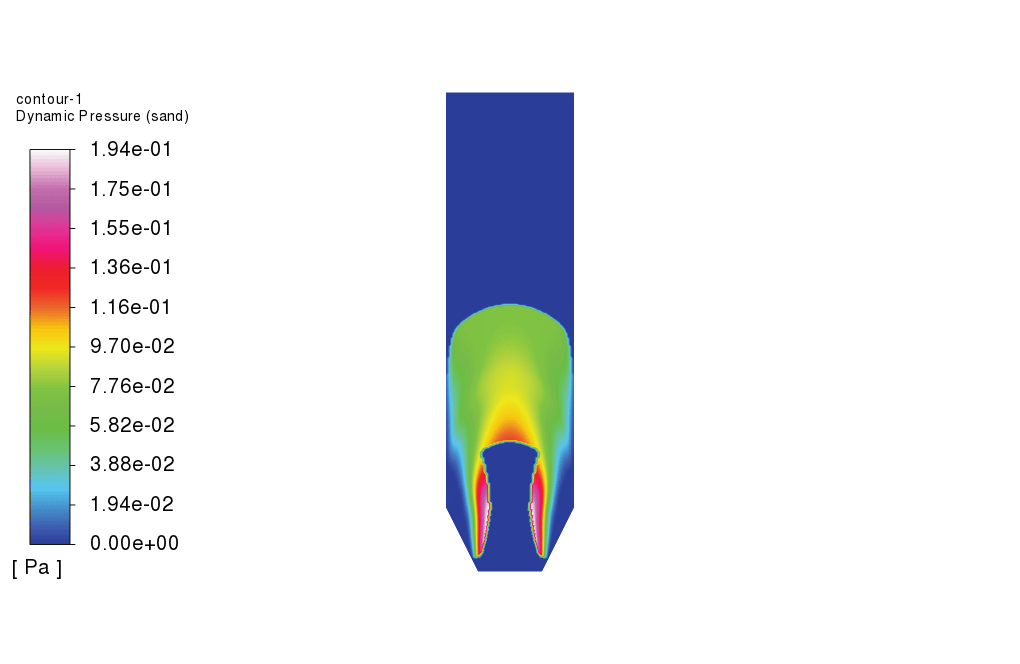
The Eulerian multiphase model was used in this project to simulate the fluidized bed bioreactor. Granular sub-model was activated and phase property model was used to obtain the granular temperature. Drag and virtual mass forces were also enabled between the phase pair of air and silicon (sand), while setting the value of restitution coefficient between the silicon particles equal to 0.9.
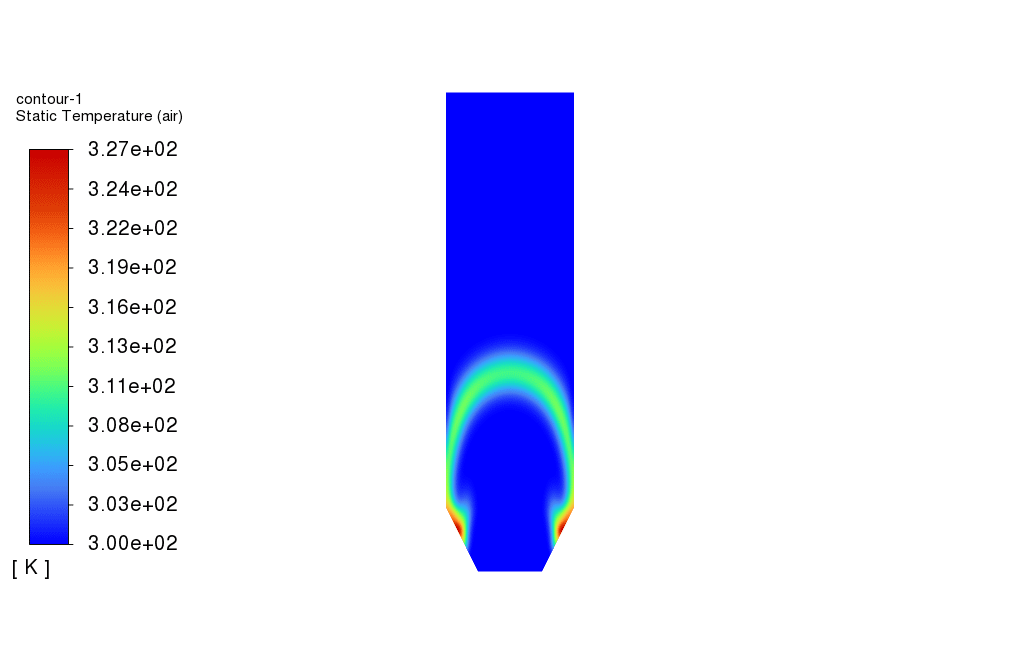
Also, Ranz-Marshall model is used to calculate the heat transfer between the air and silicon particles which can calculate the new temperature of each phase after their interactions.
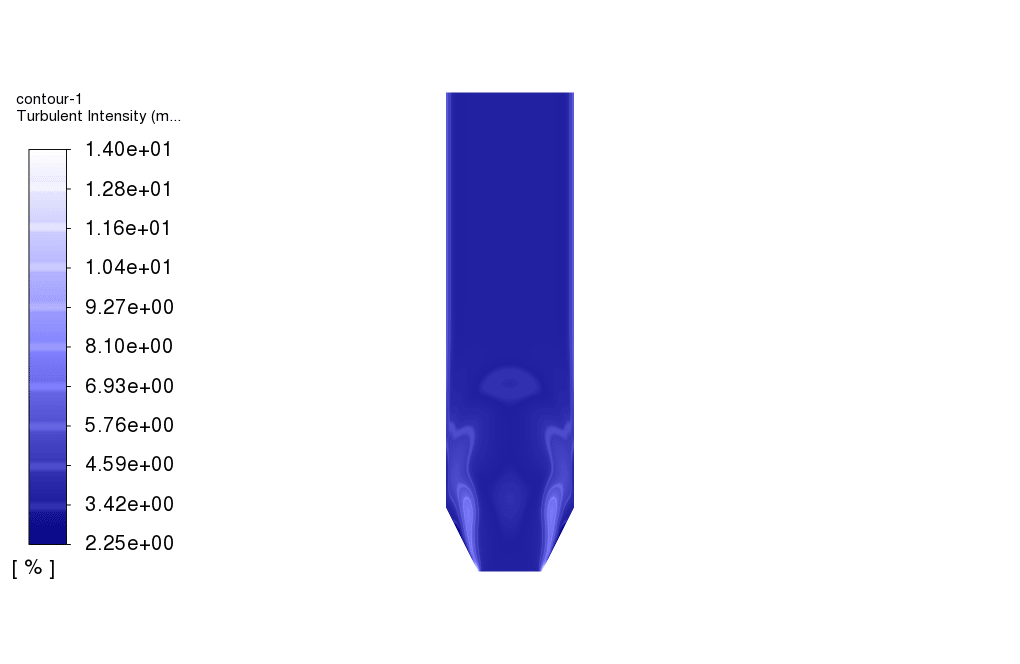
Furthermore, energy equation is enabled to calculate the temperature change inside the domain. In addition, standard k-epsilon model is used to solve for the turbulent fluid equations. The present study is performed in transient format and 2D planar model is used to simulate the problem inside a two-dimensional domain.
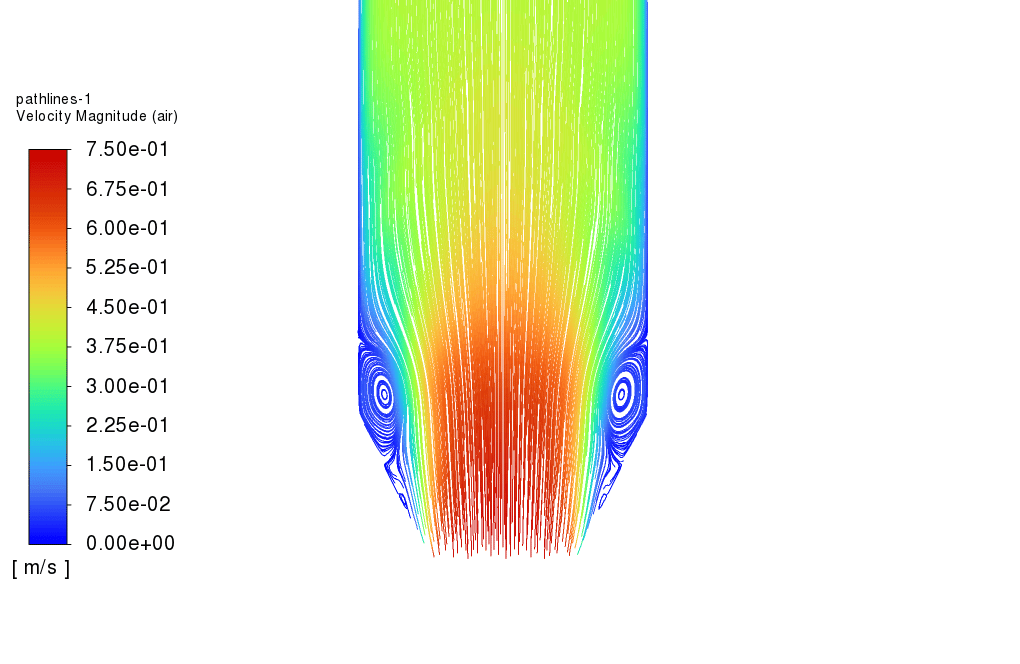
Gravity is also enabled to observe the motion of particle. As the air blows under them lifting the silicon particles inside the bio-reactor.
Conclusion
Finally, as shown in the obtained results, due to the air blowing under the silicon particles, they tend to move upward because of the fact that the exerted force on the silicon particles balances their weight. Also, similar to what occurs inside a real bio-reactor, the temperature of the fluid changes. This temperature change helps the reactions to occur faster (considering the fact that catalysts work better in higher temperatures).
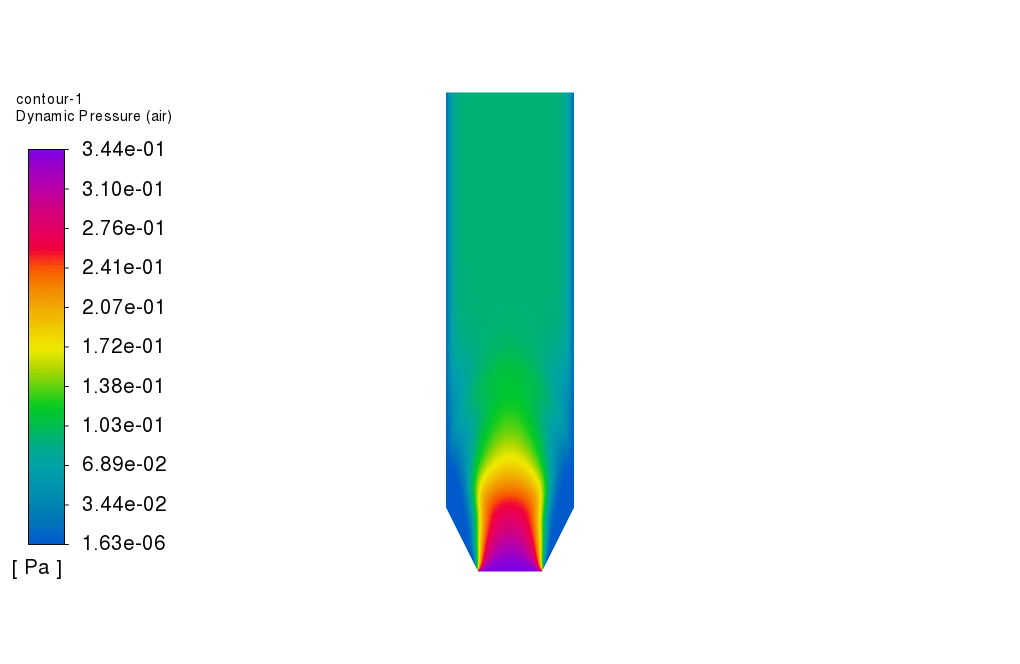
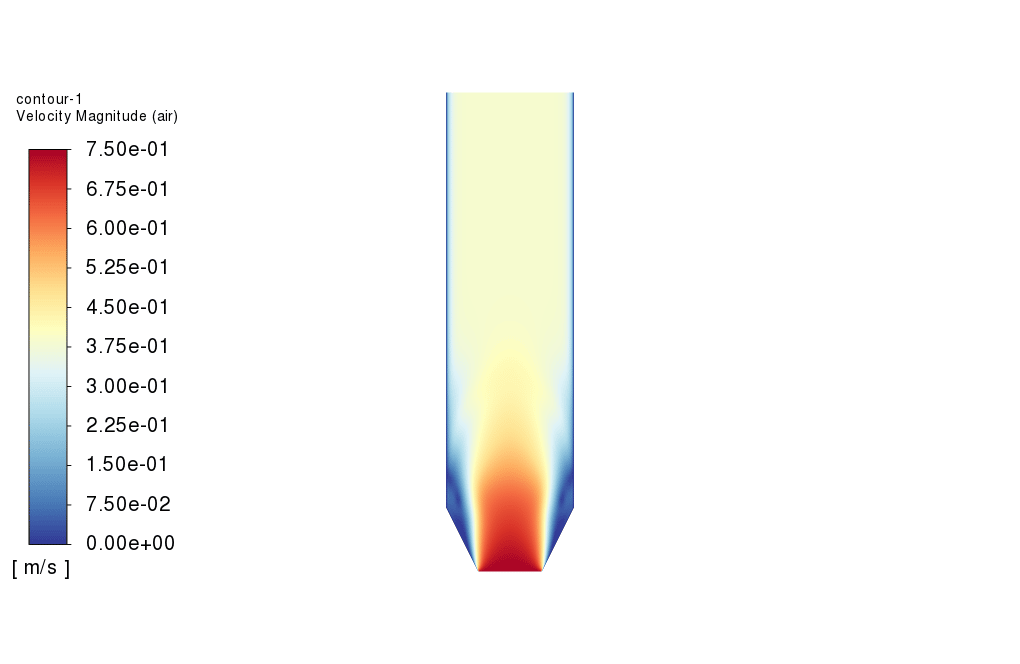

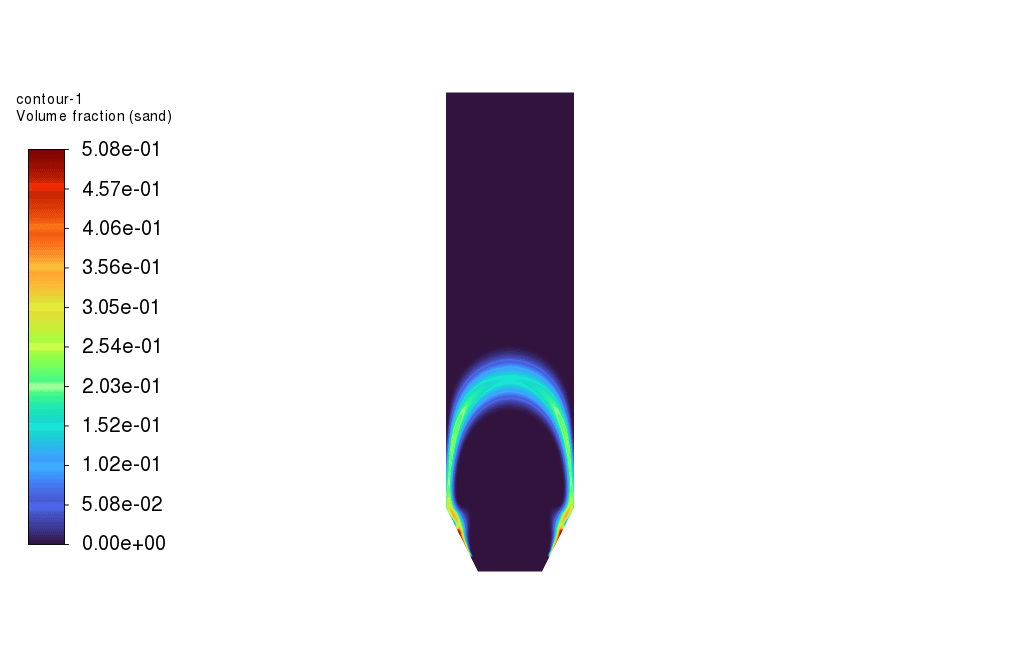
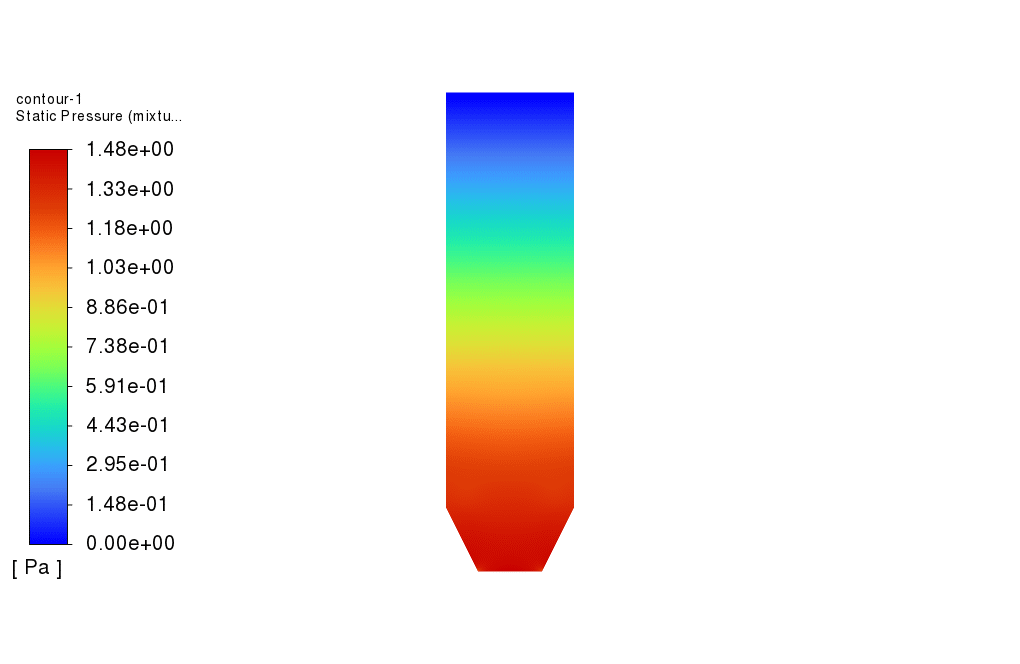
Miss Georgianna Cummerata –
I absolutely loved the clear explanation this chapter provided on the fluidization process in the FBR. The visualizations helped me grasp the complex interactions between the air and silicon particles as well as the heat transfer occurring as a result of those interactions. Thank you MR CFD for such an informative module!
MR CFD Support –
Thank you for your kind words! We are thrilled to hear that you found the chapter on fluidized bed bio-reactors both informative and visually helpful. It is our pleasure to provide quality educational materials that help our customers understand complex processes in an easy-to-follow manner. Your positive feedback is greatly appreciated, and we look forward to assisting you with more learning products in the future.
Prof. Polly Fritsch Jr. –
I found the application of heat transfer calculations between air and silicon particles through the Ranz-Marshall model particularly fascinating in your Fluidized Bed Bio-Reactor simulation product.
MR CFD Support –
Thank you for your appreciation! It’s great to hear that you find the application of the Ranz-Marshall model in simulating heat transfer intriguing. Our aim is to offer detailed and realistic CFD training that aids in understanding complex processes in bioreactor systems. If you have any more feedback or need further information, feel free to reach out!
Miss Katlynn Ruecker –
This course seems very thorough. The interplay between fluid dynamics and heat transfer in the FBR model depicted sounds fascinating. If someone has limited experience with ANSYS Fluent but a good foundation in chemical engineering, would they be able to follow along and benefit fully from this chapter?
MR CFD Support –
Absolutely! This training chapter is designed to build on your chemical engineering foundation and guide you through the specific applications of ANSYS Fluent in simulating fluidized bed reactors. With structured lessons and comprehensive explanations of the Eulerian multiphase model, even those with limited ANSYS experience can grasp the necessary concepts and apply them effectively.
Cristal Hammes –
The tutorial was exceptionally clear and comprehensive. Thanks for providing such a detailed look into fluidized bed bioreactors and the application of the Eulerian multiphase model. The explanation of drag and virtual mass forces between phase pairs was particularly instructive.
MR CFD Support –
Thank you for your great feedback! We’re thrilled to hear that you found the Fluidized Bed Bio-Reactor ANSYS Fluent Training tutorial clear and valuable. We always strive to provide detailed and instructive content, and we’re glad it was beneficial to you. If you have any more questions or need further assistance, feel free to reach out!
Mrs. Queen O’Conner I –
I’m absolutely thrilled with the clear demonstrations in the ‘Fluidized Bed Bio-Reactor ANSYS Fluent Training’. The intricate details on how fluidization occurs in industrial applications were well explained and easy to grasp. Their use of the Eulerian model to depict this phenomenon was enlightening and enhanced my understanding immensely!
MR CFD Support –
Thank you for your positive feedback on the Fluidized Bed Bio-Reactor ANSYS Fluent Training! We’re pleased to hear that the demonstrations were clear and helpful in understanding fluidization in industrial applications. It’s great to know that our training materials have contributed to enhancing your knowledge. We appreciate your kind words and look forward to providing you with more quality learning material.
Regan Metz –
The training on fluidized bed bioreactors was incredible. Learned so much about FBRs and how to model them in ANSYS Fluent. The step-by-step guide on setting up an Eulerian multiphase model with granular temperature was spot on. Can’t wait to implement these techniques in my own simulations!
MR CFD Support –
It’s fantastic to hear how beneficial our Fluidized Bed Bio-Reactor training was for you. Your excitement about applying these techniques in your simulations is precisely the outcome we strive for with our course material. Thank you for your positive feedback!
Dr. Ewald Waelchi IV –
The FBR training was very enlightening! The interplay between airflow and granular silicon movement improved my understanding of fluidization—a tough topic simplified excellently through simulation. Your course structure provided clarity every step of the way, making complex concepts accessible.
MR CFD Support –
Thank you so much for your kind words! We’re thrilled to hear that our training on fluidized bed bio-reactors has clarified a complex topic for you and enhanced your understanding. Our goal is always to provide educational resources that simplify and explain intricate concepts in an accessible manner. We appreciate you taking the time to share your positive learning experience with our product!
Dr. Jalen Friesen –
This comprehensive overview was extremely helpful! The details about using the Eulerian multiphase model and granular temperature considerations shed light on the complexities of FBRs. The element of temperature’s role in enhancing reaction speeds was particularly enlightening.
MR CFD Support –
Thank you so much for your positive feedback! We’re happy to hear that our training on Fluidized Bed Bio-Reactors was useful and provided you with a clear understanding of the subject. If you have any further questions or need more insights, please feel free to reach out.
Mrs. Cortney Homenick –
The training on Fluidized Bed Bio-Reactor sounds exceptionally informative. I can tell a lot of precision has gone into both designing the curriculum and research behind it. This definitely enhanced my understanding of Eulerian Multiphase Models.
MR CFD Support –
Thank you for your kind words! We are delighted that our training on the Fluidized Bed Bio-Reactor with the Eulerian Multiphase Model has contributed significantly to your understanding. Your feedback is much appreciated, and we’re here to provide any further assistance should you require it.
Lily Bogisich –
I just completed the Fluidized Bed Bio-Reactor ANSYS Fluent Training, and I was very impressed! The course was insightful and helped me understand complex concepts in an accessible way. I appreciate the combination of theory and practical exercises which solidified my grasp on the Eulerian multiphase model.
MR CFD Support –
Thank you for your positive feedback! We’re glad to hear that the course was helpful and that you enjoyed the balance between theory and practical application. If you have any further questions or need assistance with other CFD topics, feel free to reach out.
Rodrick Jacobs –
Wonderful learning experience! The clarity of concepts on the fluidized bed bio-reactor using the Eulerian multiphase model was impressive. The incorporation of the granular sub-model with phase property models added to the simulation’s realism. Understanding the interaction between different phases, particularly how drag and virtual mass forces impact them, was vital for my work in the biomedical field. I’m extremely satisfied with how the training covered the settings for restitution coefficients and heat transfer models—it was spot on with industry applications!
MR CFD Support –
We’re thrilled to hear about your positive experience with our Fluidized Bed Bio-Reactor ANSYS Fluent Training! Our goal is always to provide comprehensive and realistic learning material tailored to a variety of industry applications, and it’s fantastic to know we’ve been successful in your case. We appreciate your feedback, and we hope our training continues to support your contributions to the biomedical field. Thank you for choosing MR CFD for your learning journey!
Jamir Brekke –
I’m really impressed with how realistic the simulation results are from the Fluidized Bed Bio-Reactor Project. Understanding how fluidization occurs and seeing it in action helps to grasp the mechanics at play in such reactions. It makes the process easier to visualize and comprehend for industrial applications.
MR CFD Support –
Thank you for your kind words! We’re delighted to hear that our simulation project has effectively demonstrated the principles of fluidization and delivered realistic results. Your understanding and appreciation of the simulation help us in our mission to bridge the gap between complex theoretical knowledge and practical application. If you require any further information or assistance, please don’t hesitate to reach out to us.
Pierce Harvey –
The training on the Fluidized Bed Bio-Reactor using ANSYS Fluent looks comprehensive. The detailed methodology combining granular flow with heat transfer presents an interesting learning experience. I would love to know more about how industrial applications can benefit from this course.
MR CFD Support –
Thank you for your interest in our Fluidized Bed Bio-Reactor ANSYS Fluent Training! We’re glad to hear that the methodology aspect of the training caught your attention. This course is designed to help professionals in the biomedical and food industries understand the dynamics of multiphase reactions and granular flow within a reactor. By mastering these concepts, one can optimize processes such as waste treatment, chemical manufacturing, and pharmaceutical production, enhancing efficiency and reducing costs. If you implement these techniques, your company could see a significant improvement in reaction times, energy efficiency, and overall product quality. Thank you once again for choosing MR CFD for your learning journey!
Mr. Clair Halvorson –
I am thoroughly impressed with the level of detail that the Fluidized Bed Bio-Reactor ANSYS Fluent Training provides, especially regarding the implementation and benefits of the Eulerian multiphase model. The step-by-step methodology explains complex concepts in a concise manner, making it easier to replicate in professional scenarios.
MR CFD Support –
Thank you for your positive feedback! We are delighted to hear that our training material met your expectations and was helpful in explaining the intricacies of the Eulerian multiphase model. Your satisfaction with the product is our top priority. If you have any further inquiries or need additional assistance, please feel free to reach out.
Mrs. Martina Rippin III –
I was absolutely fascinated by the explanations on how the fluidized bed bio-reactor operates and the CFD analysis performed with the Eulerian multiphase model. The application across multiple industries like biomedical and food is equally impressive.
MR CFD Support –
Thank you for your kind words! We’re delighted that you’ve found the explanations intriguing and the application of the fluidized bed bio-reactor across different industries informative. It’s our pleasure to provide high-quality educational materials that not only explain the theory but also show practical analysis examples. If you ever have any questions or need further clarifications, feel free to reach out!
Dr. Lurline Weimann IV –
I completed the training on the Fluidized Bed Bio-Reactor simulation using the Eulerian Multiphase Model and I’m thoroughly impressed! The hands-on approach to setting up and running the simulation has deepened my understanding of fluid dynamics in bioreactors. The visualization of air and granular flow within the reactor gave me a remarkable insight into the real-time applications in the industry. Excellent course layout and top-notch instructions!
MR CFD Support –
Thank you for your positive feedback! We’re delighted to hear that you found the Fluidized Bed Bio-Reactor training to be useful and intuitive. It’s our goal to provide a learning experience that enriches understanding and offers practical insights into complex simulations. Your appreciation means a lot to us, and we are thrilled to have contributed to your knowledge in fluid dynamics and its applications. Keep an eye out for more advanced courses and don’t hesitate to reach out if you need further assistance in your learning journey!
Stephen Olson PhD –
This training seems comprehensive. I’m curious if the study included the effects of temperature changes on the effectiveness of the catalyst within the reactor, or was it solely focused on the fluid dynamics and heat transfer aspects?
MR CFD Support –
In this specific training encompassing fluidized bed bioreactors, the main focus is to offer an understanding of the fluid dynamics and heat transfer facets using the Eulerian multiphase model. Though the temperature changes are accounted for as they pertain to the general behavior and reactions within the reactor, the specific effectiveness of catalysts under varying temperatures might not be the core emphasis. For such specialized insights into catalyst effectiveness, additional simulations or enhanced scopes of study might be recommended. Should this area be of interest, exploring more targeted analyses or courses that jointly consider reaction kinetics and thermodynamics could prove beneficial.
Sherman Pfeffer Jr. –
The detailed simulation of the fluidized bed bio-reactor fascinates me, specifically how the FBR handles the heat transfer and particle interaction. The granular temperature modeling and the use of the Ranz-Marshall model are particularly interesting. This seems quite useful for applications in the biomedical and food industries, where precision is crucial.
MR CFD Support –
Thank you for your kind words! We are thrilled that you find our Fluidized Bed Bio-Reactor ANSYS Fluent Training insightful. Understanding heat transfer and particle interactions is critical for optimizing FBR processes, and we’re glad the course content is meeting those real-world industry needs. Your feedback is greatly appreciated!