Droplet Collapse on a Wall with/without Shear Stress, Ansys Fluent Training
$100.00 Student Discount
In this project, the water droplet collapse on the wall with/without shear stress has been simulated and the results of this simulation have been investigated.
Click on Add To Cart and obtain the Geometry file, Mesh file, and a Comprehensive ANSYS Fluent Training Video.To Order Your Project or benefit from a CFD consultation, contact our experts via email ([email protected]), online support tab, or WhatsApp at +44 7443 197273.
There are some Free Products to check our service quality.
If you want the training video in another language instead of English, ask it via [email protected] after you buy the product.
Description
Project Description
In this project, Ansys Fluent software has been used for numerical simulation of water droplet collapse on the wall in two modes of non-slip condition and shear stress condition. The VOF model has been used to simulate and solve the two-phase flow field equations.
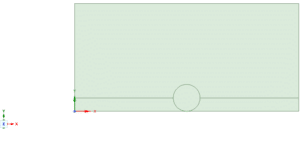
Geometry & Mesh
The 2-D geometry of the present model is generated using Spaceclaim software. The length of the computational area is 25 mm and its height is 12.5 mm.
The meshing of the present model has been done using Ansys Meshing software. The mesh type is unstructured in all of the computational domains, and the element number is equal to 53440.
Droplet Motion CFD Simulation Settings
We consider several assumptions to simulate the present model:
- Due to the incompressibility of the flow, the pressure-based solver method has been selected.
- The simulation is transient.
- The gravity effect is considered equal to -9.81 m.s-2 on Y-axis
The Laminar viscous model has been used to solve the flow field equations, and the pressure-velocity coupling scheme is SIMPLE. The second-order upwind discretization method has been used for momentum and PRESTO! For the pressure discretization.
The following tables represent a summary of the defining steps of the problem in this project and its solution:
| Models | ||
| Multiphase | ||
| Homogeneous model | Volume of fluid | |
| Number of Eulerian phases | 2(air& water) | |
| Interface modeling | Sharp
Interfacial |
|
| Formulation | explicit | |
| Body force formulation | Implicit body force | |
| Viscous | Laminar | |
| Material Properties | |
| Air | |
| Density | 1.225 |
| viscosity | 1.7894e-05 |
| water-liquid | |
| Density | 998.2 |
| viscosity | 0.001003 |
| Methods | ||
| Pressure-Velocity Coupling | SIMPLE | |
| Pressure | PRESTO! | |
| Momentum | Second-order upwind | |
| Volume fraction | Compressive | |
| Initialization | ||
| Initialization methods | Standard | |
| Patch | Phase | Phase2 |
| Variable | Volume Fraction | |
| Registers to patch | Region_0 | |
| Value | 1 | |
| Run calculation | ||
| Time advancement | Type | adaptive |
| Parameters | ||
| Initial time step size | 0.0003 | |
| Settings | Minimum time step size | 0.0003 |
| Maximum time step size | 0.0003 | |
| Time step size | 180 | |
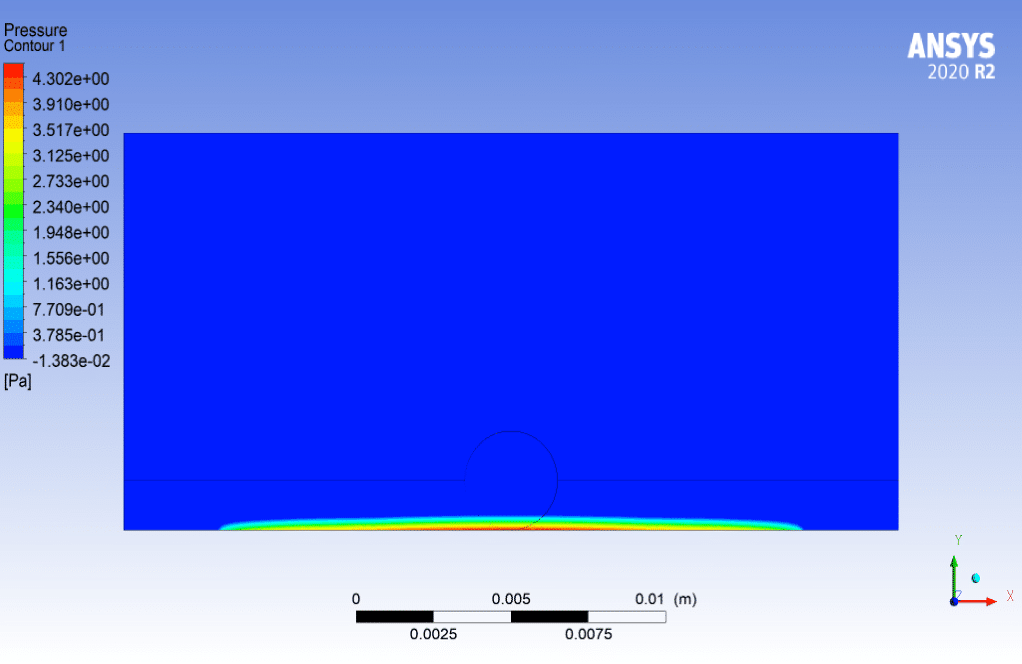
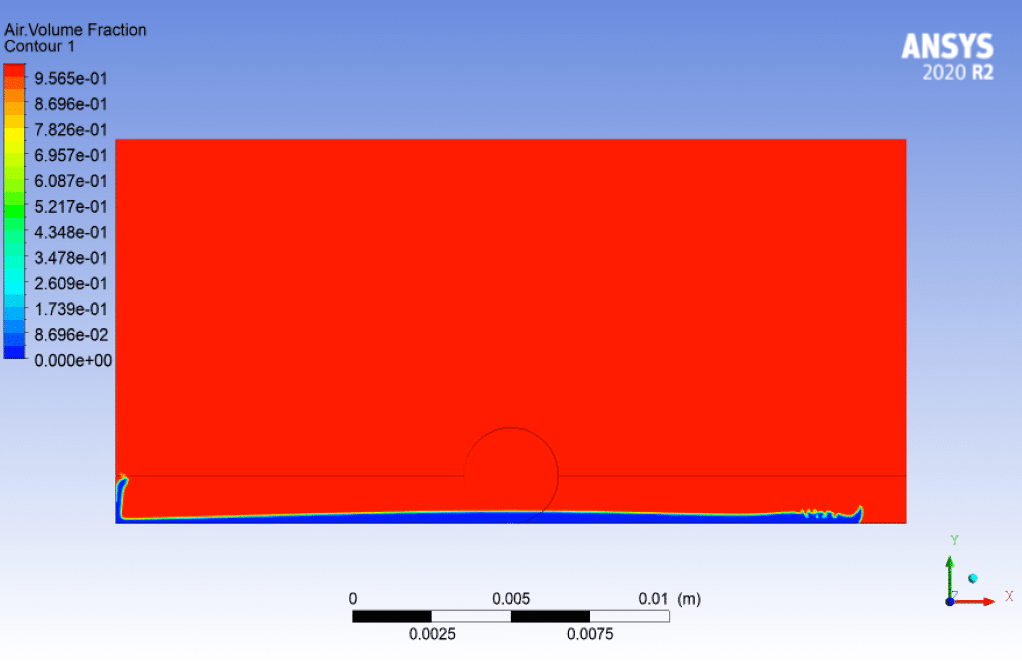
Droplet Motion Results
In this simulation, the free surface of the water droplet is investigated in two modes. The drop is primarily crushed, and the surface’s rate of dispersion and wetting has increased, which intuitively, we expect the same behavior from the movement of the fluid in the presence of slip.
[/vc_column_text]





Mr. Kiley Hamill V –
What type of droplet sizes are being used in the simulations, and how is the droplet placed in the computational domain?
MR CFD Support –
In the given simulation, the exact size of the droplet is not detailed, however, typically droplets in such simulations are in the range of millimeters. The droplet is placed within the computational domain using the ‘patch’ function in the initialization step defining a volume of fluid (Phase2) initially as 1 in the Region_0, which represents the initial shape and location of the droplet on the wall.
Miss Name Reichel –
Fantastic learning resource for droplet dynamics! The detailed breakdown of theory, simulation setup, and results allowed me to grasp a complex topic thoroughly. The use of both non-slip and shear stress conditions offered a comprehensive understanding of different scenarios.
MR CFD Support –
Thank you for the glowing review! We’re thrilled to hear our training material on droplet dynamics was helpful in enhancing your understanding. Your success is our top priority. If you have any further queries or need additional assistance with your learning journey, don’t hesitate to reach out!
Anna B. –
The “Minimum time step size” and the “Maximum time step size” are the same. So why did you use adaptive time advancement?
Myrna McDermott –
I’m really impressed with how comprehensive the Project Description is! This must have been a meticulous process.
MR CFD Support –
Thank you for recognizing the detail and effort put into the simulation process. We are glad you appreciate the thoroughness of the Project Description. Your positive feedback motivates us to continue delivering detailed and practical training materials.
Mrs. Kali Stehr III –
I’m very impressed with the level of detail in this CFD analysis of droplet motion. Has the team considered the use of different wall materials to observe the effects on droplet dynamics?
MR CFD Support –
Thank you for your keen observation and compliment on the droplet motion simulation project. We are glad to hear that you appreciate the detailed analysis. Regarding your question, for the specific project you mentioned, we focused on the dynamics of a water droplet collapsing on a wall under non-slip conditions and shear stress without varying wall materials. However, the simulation can potentially be adapted to include different wall material properties to observe how they affect droplet behavior, such as wettability, contact angles, and surface tension effects. If you have any particular material in mind or a specific application you’re interested in, let us know, and we can explore customization options for future simulations.
Tressa Braun –
Could you please clarify if the effect of temperature variation was considered in the modelling of droplet collapse?
MR CFD Support –
Temperature variation effects were not explicitly included in the project description for the water droplet collapse simulation. The simulation focused on using a Laminar viscous model for flow field equations and the VOF model for two-phase flow without mention of thermal effects.
Dr. Emilia Auer –
I appreciated the walkthrough of various simulation settings. Could I expect to simulate similar results on my system or are there any system-specific steps taken that weren’t mentioned in the description?
MR CFD Support –
Thank you for your kind words! We aim to make the simulation as system-independent as possible. All model setup and configuration steps are mentioned in the description. In ANSYS Fluent, simulation results are usually consistent across different systems when the same settings and steps are followed. Do ensure your system meets the specifications required by the ANSYS software for optimal performance.
Karley Leannon –
I am thrilled with how clear the simulation results were. The visuals really helped me understand the droplet collapse dynamics in both non-slip and shear stress conditions.
MR CFD Support –
Thank you for your positive feedback. We’re glad to hear that our visuals were effective in conveying the complex dynamics of droplet collapse on a surface. Your understanding of the simulation is exactly what we aim for with our instructional materials!
Darian Schroeder –
The explanation of droplet collapse is very intriguing. Could you share more about how the wetting characteristics changed with and without the shear stress conditions?
MR CFD Support –
The wetting characteristics in the droplet collapse simulation vary significantly between non-slip and shear stress conditions. Without the shear stress, the droplet spreads axially along the wall, resulting in a classic wetting phenomenon. However, with shear stress applied, the flow dynamics are altered, causing a distortion in the drop shape and an increased rate of spread. The shear force acts tangentially to the droplet’s surface, contributing to a different wetting pattern and an asymmetric spreading of the droplet.
Ivah Wuckert MD –
The training material seems very thorough! Did the simulation provide any insights on the potential applications of studying droplet collapse in industrial processes?
MR CFD Support –
Yes, indeed! The simulation offers valuable insights into the dynamics of liquid droplets impacting surfaces, which is vital for a range of industrial applications such as inkjet printing, coating processes, and cooling systems. Understanding droplet behavior can help improve efficiency and quality in these areas.
Mrs. Brandyn Rutherford I –
I’m quite impressed with the level of detail in the simulation for the water droplet collapse. Can this model be used for completely different liquids or just for water? I am considering adapting the procedure to simulate a different fluid behavior.
MR CFD Support –
Thank you for your kind words! Yes, the model can be adapted to simulate different liquids by changing the material properties such as density and viscosity to match the characteristics of the new fluid. Make sure to also adjust the boundary conditions and possibly the solver settings if the fluid dynamics significantly differ from water.
Magdalen Carroll –
This CFD simulation’s attention to detail is impressive. The adaptive time step feature and explicit VOF model should provide a very accurate depiction of the droplet collapse and spreading. Great work on this training simulation!
MR CFD Support –
We are thrilled to hear that you are satisfied with the accuracy and detail provided in our Droplet Collapse simulation training module. Our team strive to offer high-quality educational simulations to enhance learning. Thank you for your positive feedback, it’s greatly appreciated!
Timmy Grant –
This CFD training module appears to be extremely informative. I was particularly impressed by the detailed meshing and assumptions used to realistically model the water droplet collapse. The use of VOF model shows MR CFD’s dedication to providing comprehensive simulation scenarios.
MR CFD Support –
Thank you for your kind feedback! We are thrilled to hear that you found our training material on the droplet collapse simulation informative and realistic. At MR CFD, we continuously strive to deliver detailed and thorough education on various CFD concepts. We’re glad you appreciated the efforts on meshing and the implementation of the VOF model.