Accumulation of Particles in Elbow Bend by DDPM
$160.00 Student Discount
- The problem numerically simulates a simple elbow bend using ANSYS Fluent software.
- We design the 3-D model with CAD software.
- We mesh the model with ANSYS Meshing software.
- The mesh type is Structured, and the element number equals 150624.
- This project is performed in an unsteady state (transient).
- We use the DDPM (Dense-Discrete-Phase-Method) in this project.
To Order Your Project or benefit from a CFD consultation, contact our experts via email ([email protected]), online support tab, or WhatsApp at +44 7443 197273.
There are some Free Products to check our service quality.
If you want the training video in another language instead of English, ask it via [email protected] after you buy the product.
Description
Accumulation of Particles in Elbow Bend by DDPM, ANSYS Fluent CFD Simulation Training
We have simulated a simple elbow bend in the current project. However, the fast injection would result in a dense accumulation of particles. Our computational domain comprises a 25 [mm] annulus of the air and particle mass flow of 4.34×10-7 [kg/s].
The diameter of particles considered is 10.06×10-12 [m] with a velocity magnitude of 0.09625 [m/s]. Both phases were considered unsteady, and the injection time for the particle was considered equal at each flow time-step of 0.005 [s].
As a numerical study, the initial step toward modeling is the production of the CAD geometry. We consider the blue face as the inlet of the domain while the red face is the outlet.
For the current problem, we generate a mesh count of 150,624 elements to represent half of the domain and use symmetry at the middle surface of the domain.
Regarding the mesh quality, the maximum skewness of 0.05 is low due to being a structured mesh. Finally, we performed the meshing operation via ANSYS-Meshing software.
CFD Methodology
While the DPM strategy for CFD solutions proved to be an excellent method to calculate flow particle-flow studies, this approach cannot provide reliable answers for dense particle simulations. The DDPM (Dense-Discrete-Phase-Method) is mainly employed in CFD projects to remove this issue.
Therefore, a dense DDPM model is employed. To study the current problem, one must solve the flow equations in the differential form. Also, we assume the incompressible and turbulent condition inside the elbow geometry since the particle-flow interactions are more likely to create a turbulent flow condition.
Also, we have employed a Realizable k-epsilon model with a Menter-Lechner wall function to account for our boundary layer. Also, a Transient solver is applied.
In the current study, we have followed the convergence up to a tight residual value of 10-10. By adding more particles to the domain, the necessity to improve the convergence became more critical due to the higher impact of the source term.
Also, the mass average of the source term in the y-direction was monitored during the solution to ensure that each time step satisfied the required accuracy.
Accumulation of Particles in Elbow Bend Conclusion
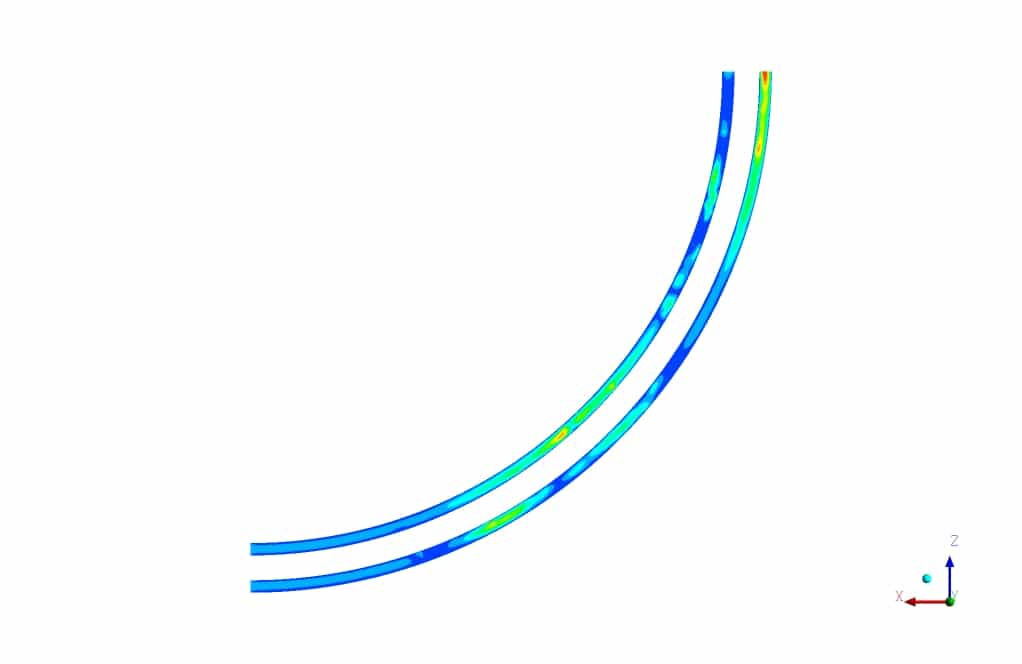
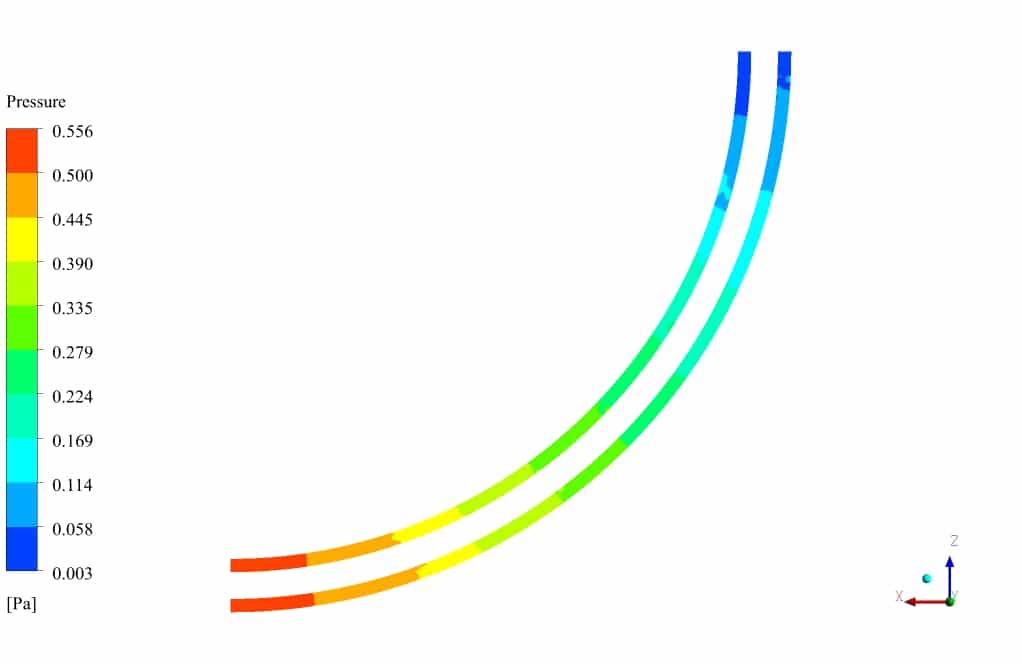
Afterward, the pressure and velocity field results are depicted for both particles and the air-fluid flow below the figures. As observed, the air velocity increases as the flow reach the bend section.
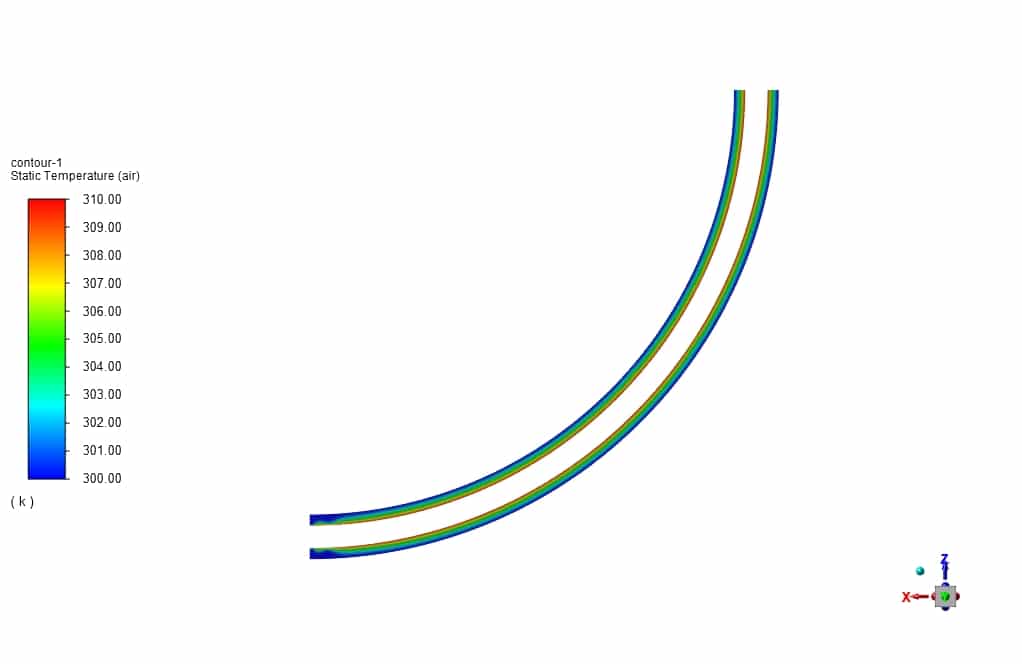
Also, the flow temperature close to the hot wall, which is the inner wall, is higher than on the other side of the pipe. Furthermore, the hot temperature diffused among fluid flow and particles over time.
Interestingly, the same results were observed from the DPM particle calculations: velocity and pressure were higher at the exact locations. Additionally, the streamlines imply that several vorticities were formed close to the bend location, which is usually interesting for erosion simulations.



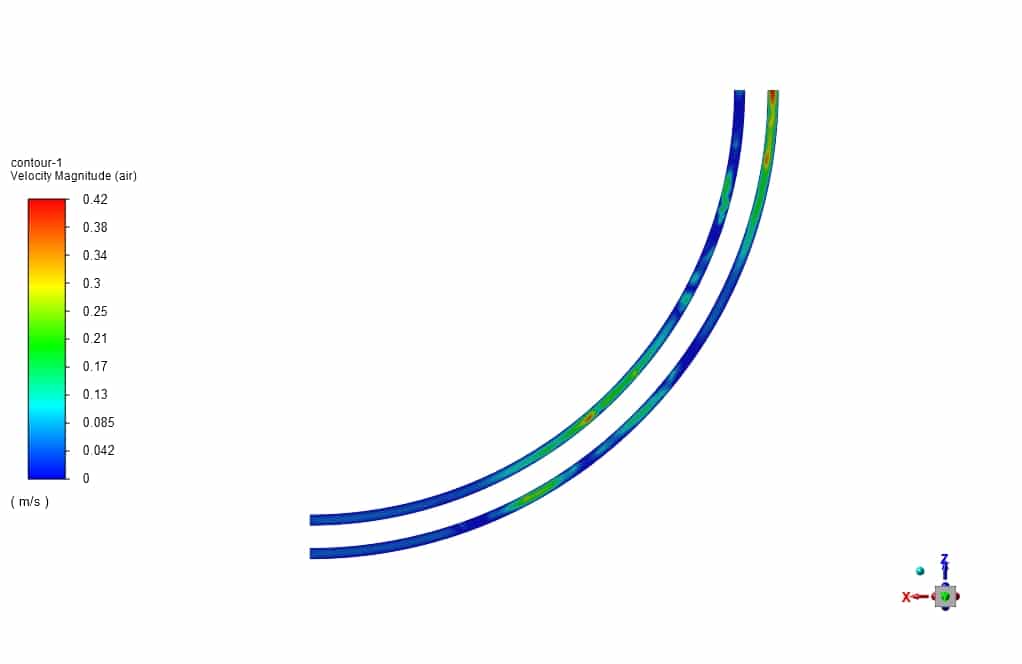





Reviews
There are no reviews yet.