Horizontal Axis Wind Turbine (HAWT) Aerodynamic, ANSYS Fluent Training

Description

Description

This simulation is about horizontal axis wind turbine (HAWT) aerodynamics via ANSYS Fluent software. We perform this CFD project and investigate it by CFD analysis.

Standard Horizontal Axis Wind Turbine (HAWT) is becoming increasingly critical in wind power generation. Fortunately, it is known that HAWTs have higher efficiency compared to VAWTs. Thus, they have been employed in open fields and can produce energy from the wind.

As wind tunnel experiments are expensive in terms of both costs and time, another way to study the aerodynamic behavior of the wind turbine is to use CFD. In this project, CFD has been employed to evaluate this type of turbine in this study.

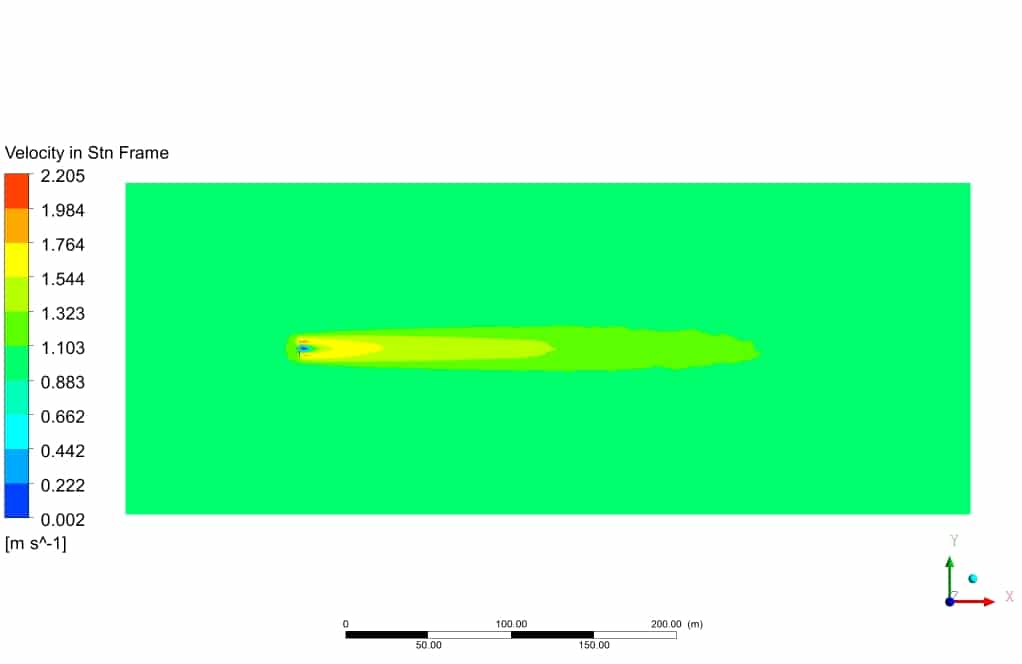
The geometry included a rotary zone for the turbine walls and a stationary zone for the rest of the domain. The inlet is considered to wind at 1 m/s, and the turbine zone rotates at 16 RPM.

An incompressible and isothermal condition is a valid assumption for the current simulation. This study aims to investigate the behavior of airflow and pressure distribution and study drag force.

The geometry of the present model is drawn by Design Modeler software. The model is then meshed by ANSYS Meshing software. The model mesh is unstructured, and 2463521 cells have been created.

Horizontal Axis Wind Turbine Method

In this simulation, the MRF (Frame Motion) option has been activated to model the rotation of the turbine. In fact, it is assumed that the fluid around the turbine blades is rotating instead of the turbine blades themselves.

The rotational velocity of this fluid is equal to the rotational velocity of the turbine. In order to do this, the MRF tool is used in Cell Zone Conditions.

Horizontal Axis Wind Turbine Conclusion

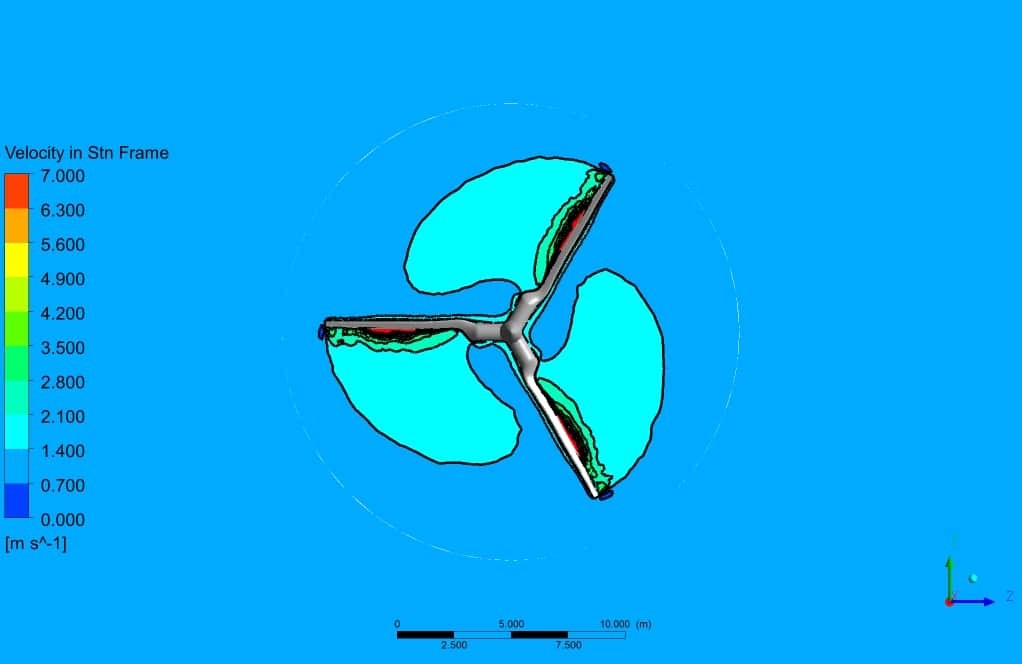
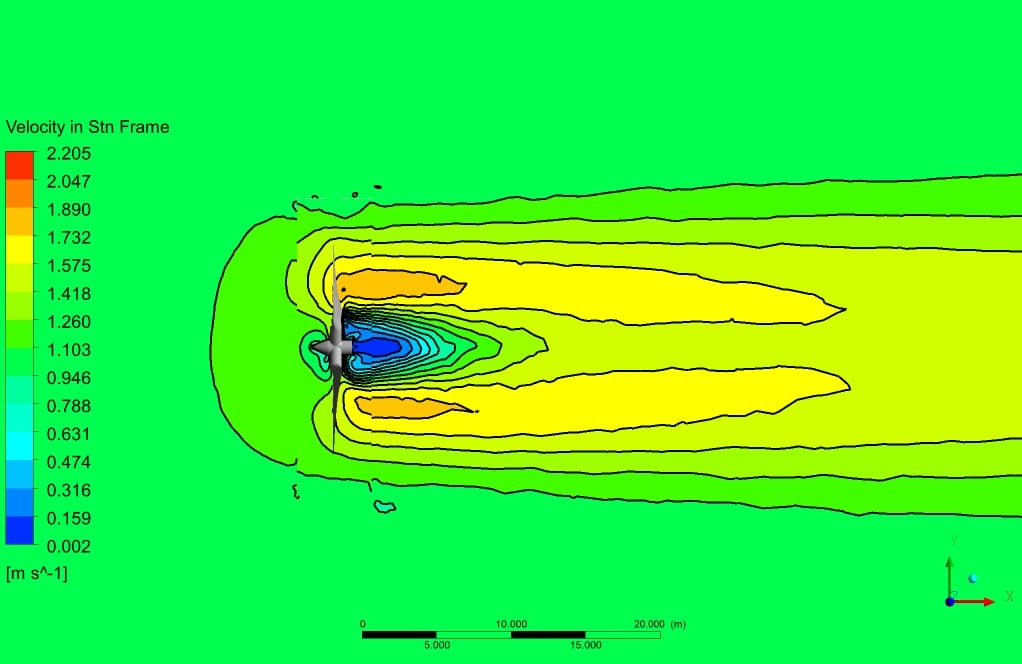
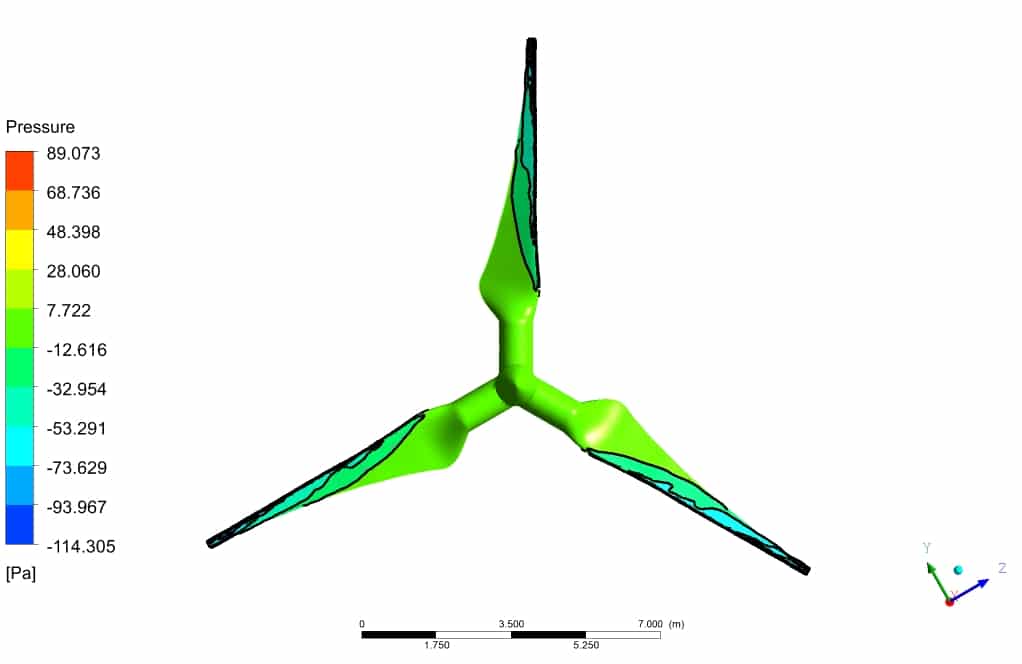
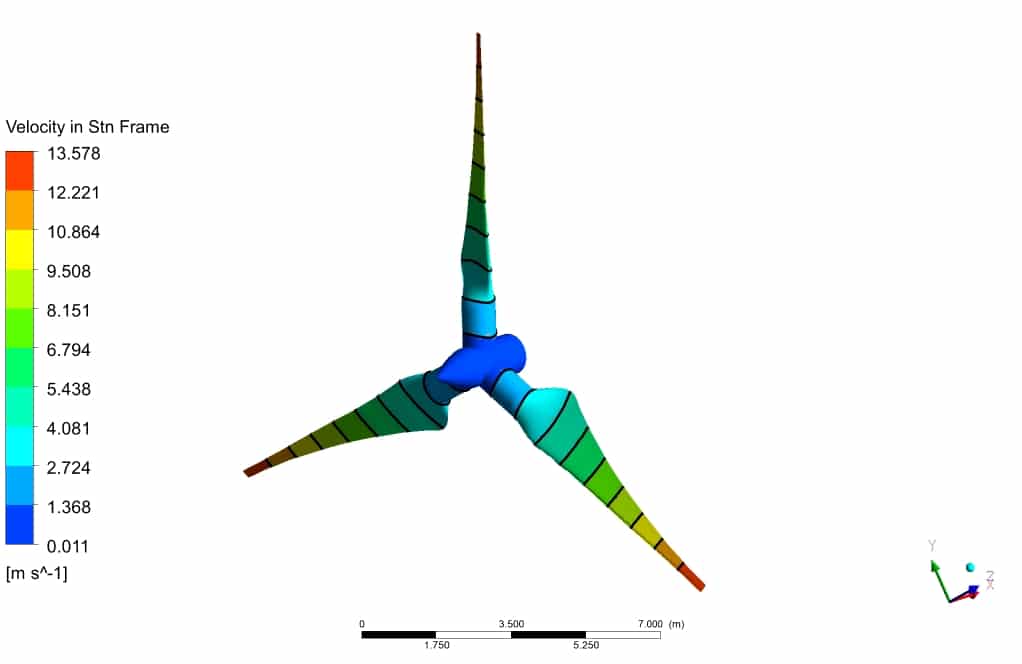
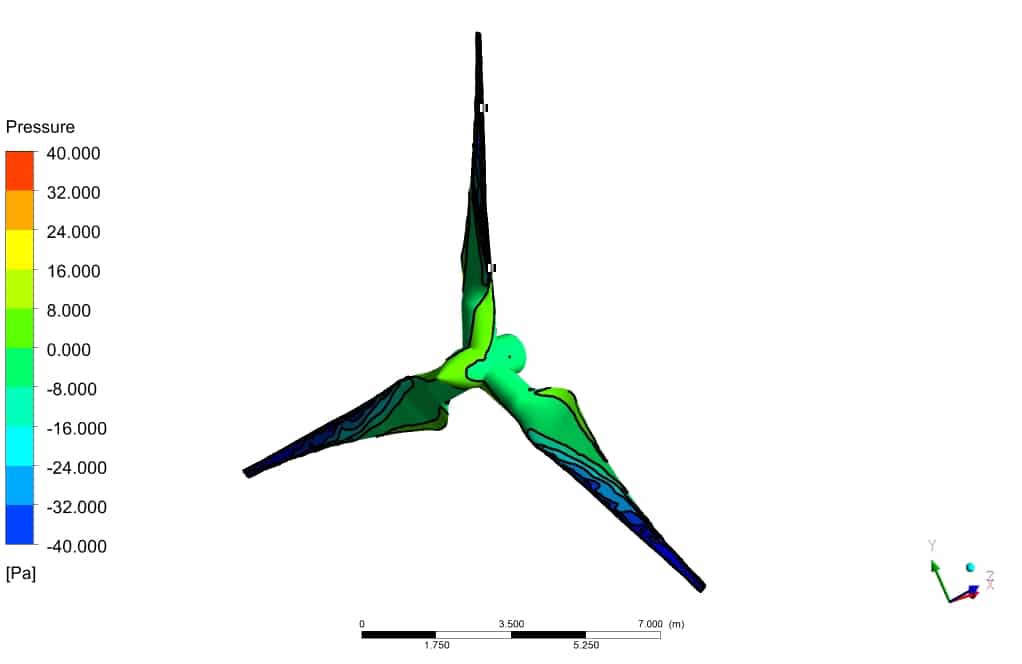
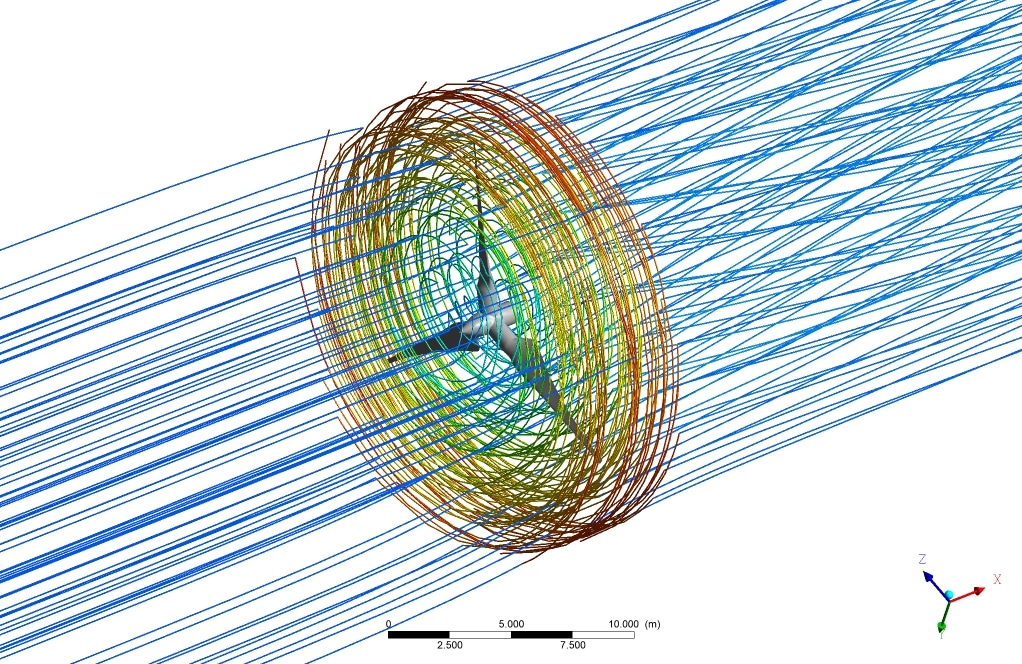
After simulation, the contours of pressure, velocity, and surface pressure are obtained. Also, the streamlines of fluid around the turbine are obtained. The streamlines illustrate the flow quality resolved in the wake section, which is the core challenge of aerodynamic simulation.

The contours show that the leading edge of the turbine wall corresponded to the lowest pressure, which is entirely logical since the velocity has the highest value on the tip of the turbine blade. Also, the velocity field adjacent to the wall of the turbine has the highest gradient.

Finally, we calculate the drag force equal to 243.63 (N), which is accurate for a 3-meter turbine with the noted specifications.