Solar Water Desalination by Species Transport, 2-D
$220.00 Student Discount
- The problem numerically simulates the Solar Water Desalination using ANSYS Fluent software.
- We design the 3-D model by the Design Modeler software.
- We mesh the model with ANSYS Meshing software.
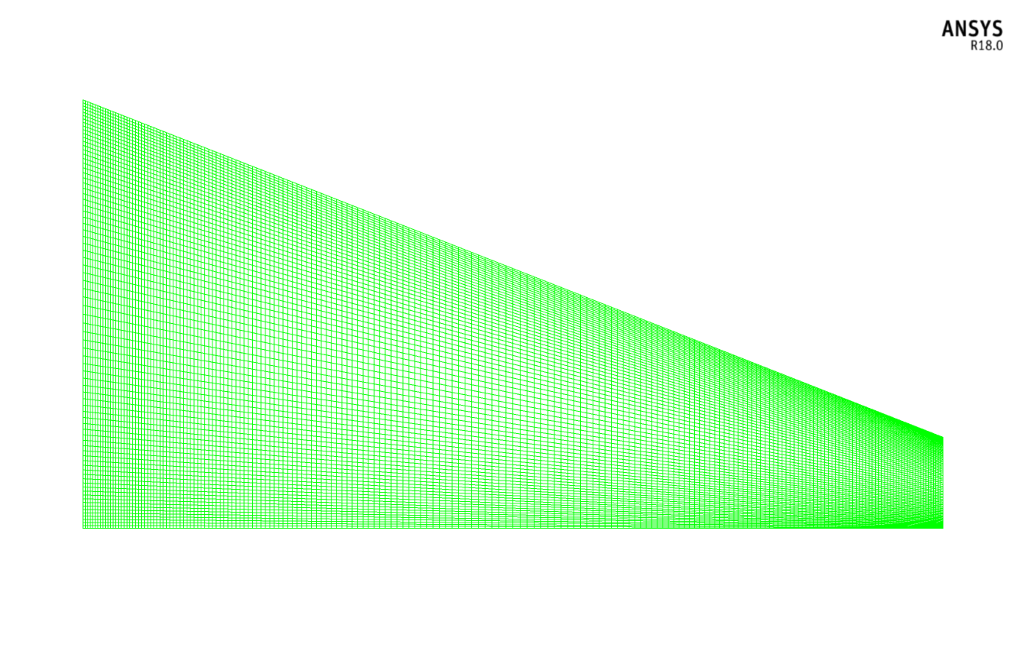
- The mesh type is Structured, and the element number equals 16000.
- We perform this simulation as unsteady (Transient).
- We use the Species Transport model to define 3 different species.
- We use a UDF to define mass transfer rate in the form of evaporation-condensation.
To Order Your Project or benefit from a CFD consultation, contact our experts via email ([email protected]), online support tab, or WhatsApp at +44 7443 197273.
There are some Free Products to check our service quality.
If you want the training video in another language instead of English, ask it via [email protected] after you buy the product.
Description
Solar Water Desalination 2-D (Species Transport) , ANSYS Fluent CFD Simulation Training
The present study investigates the performance of a solar-still thermal desalination unit by ANSYS Fluent software. The current model consists of a small chamber with a sloping glass surface at the top. The resulting vapor impacts the cold glass surface and undergoes a distillation process. Pure water from hot vapor distillation moves down the slope of the glass plate and discharges as pure water.
One of the thermal methods of water desalination is using solar desalination units. In solar water desalination, solar energy is used as a heat source and causes the evaporation process. In the functional mechanism of this type of desalination, surface evaporation occurs by solar energy. The produced vapor collides with the cold surface of the glass and converts to pure water after distillation.
Desalination Methodology
The solar heat passes through the glass to the water’s surface in the enclosure and causes surface evaporation (applying a UDF for surface evaporation).
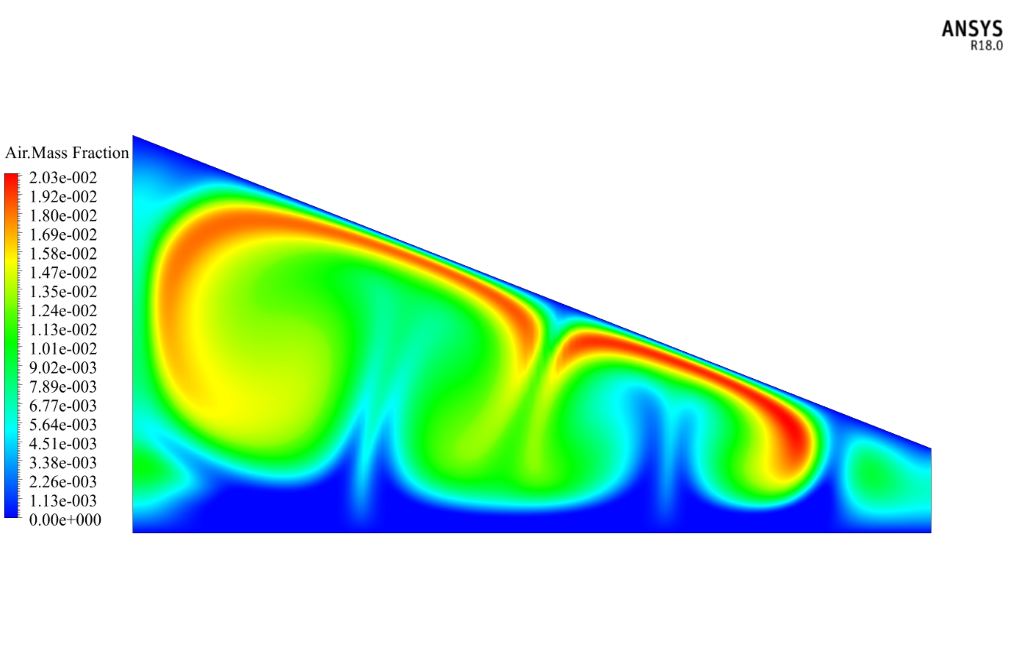
The Species Transport method is used. This method does not simulate saline water flow in the device. Unlike multiphase simulations with much heavier computations, this method assumes that there is a mixture of air and vapor inside the chamber interior.
The simulation focuses solely on the amount of vapor produced in the system. The present study aims to investigate the amount of vapor produced on the water surface and the cold surface of the sloping plate.
Also, the solver is Steady-State. The present 2-D model was designed by Design Modeler software. The geometry consists of a sloping glass surface at the top and a horizontal water plate at the bottom. A Structured mesh was performed using ANSYS Meshing software. The element number is 16,000.
Desalination Conclusion
This method assumes a mixture of air and vapor inside the chamber interior and does not simulate distilled water flow.
In fact, at the beginning of the simulation, the space inside the chamber is filled with air only; the bottom plane of the chamber is considered the surface of the water, and since the water evaporates on the surface, it is assumed that the surface consists of vapor.
It is also assumed that the sloping plate where the steam collides is composed only of vapor. So water and air mass fraction contours completely show the evaporation in the device.





Dr. Tiffany Murray IV –
Hello,
I have used MR-CFD tutorials before, which are mostly excellent. I needed this training.
Of course, please prepare training about nanofluid.
Thankful
MR CFD Support –
Hello dear, you can also go to
http://www.mr-cfd.com/shop/nanofluid-optimum-concentration-for-heat-transfer-enhancement and use this tutorial.
Marques Wolff –
Can this simulation be used for other types of solar desalination systems?
MR CFD Support –
While this simulation is specifically designed for a solar still, the underlying principles and models can be adapted for other types of solar desalination systems.
Prof. Shyanne McDermott Jr. –
Is it possible to change the design of the solar still in the simulation?
MR CFD Support –
Yes, the design parameters of the solar still can be modified in the simulation to suit your specific requirements or to optimize the design.
Shanel Baumbach –
Can this simulation model account for different weather conditions?
MR CFD Support –
Yes, the simulation can be adapted to account for various environmental conditions. However, such customizations may require additional computational resources.
Payton Muller –
Thank you Sir. I tried this but I am getting error: floating point exception after pressing calculate. Error object #f is being shown. How do I fix it?
MR CFD Support –
May be error with your meshing… Please check quality of your mesh.
Kayden Stokes V –
Please also teach about melting and solidification.
MR CFD Support –
You can prepare Solidification and Melting of PCM around a Corrugated Tube In the link below:
http://www.mr-cfd.com/shop/solidification-and-melting-of-pcm-around-a-corrugated-tube
Saige Armstrong –
Can I use this simulation for academic research?
MR CFD Support –
Yes, this simulation can be a valuable tool for academic research in the field of solar desalination. It can help in understanding the underlying phenomena and in developing more efficient desalination systems.
Prof. Eric Spencer PhD –
This CFD training product for solar water desalination appears fascinating. I was wondering, though, have any studies addressed potential solutions for increasing the efficiency of this desalination process?
MR CFD Support –
Absolutely! Various strategies can be employed to boost the performance of a solar still desalination system. This includes optimizing the chamber’s shape, improving the heat absorptivity of the water basin, using selective coatings on the glass cover, and incorporating phase change materials to store solar energy. Each technique would require specific modifications to the simulation setup, which can certainly make for an intriguing follow-up study.
Joesph Harvey Jr. –
The description tells me how the process works but doesn’t mention temperatures. What temperatures are set for the vaporization process, and what surface temperature is considered for the condensation on the glass plate?
MR CFD Support –
The simulation setup for vaporization takes into account solar energy as the heat source. A UDF (User Defined Function) could potentially define the specific temperature at which water evaporates. As for the condensation on the glass plate, it typically represents a colder surface, but the exact temperatures could vary. The simulation results would indicate the temperature gradient necessary to achieve the distillation process. Since these specifics weren’t provided, I’d recommend revising the product documentation for detailed temperature parameters.
Rebekah Berge –
What kind of simulation setup allows the solar water desalination study to avoid simulating saline water flow within the device?
MR CFD Support –
The Species Transport method is utilized in the solar water desalination study to avoid simulating saline water flow within the device. This method assumes that there is a mixture of air and vapor inside the device instead of tracking the separate phases of saline water and air.
Gardner Gerlach –
I’m fascinated by the Species Transport method used in the solar water desalination simulation. The efficiency of solar energy conversion seems crucial. Can you tell me how the evaporation rate varies with changes in solar energy intensity? Is there a direct correlation model included in the software for this purpose?
MR CFD Support –
In the solar water desalination simulation using ANSYS Fluent, the evaporation rate is directly influenced by the intensity of solar energy. To model this, a User-Defined Function (UDF) can be implemented to define the relationship between the evaporation rate and solar energy intensity, allowing you to take into account any direct correlation. This UDF will specify the heat transfer and phase change at the water surface to simulate evaporation.
Tiara VonRueden –
I completed the Solar Water Desalination training. I was thoroughly impressed by the clarity of the study and CFD simulations. Great job!
MR CFD Support –
Thank you for your kind words! We’re delighted to hear that you found the Solar Water Desalination training helpful and clear. We strive to provide the best quality simulations and appreciate your feedback!
Sammie Pfeffer Sr. –
Does this solar desalination simulation account for the temperature variations throughout the day?
MR CFD Support –
No, the described simulation assumes a steady-state solver, which means it does not account for transient thermal effects like temperature variations throughout the day. It simplifies the process by focusing on a single state, rather than changing conditions over time.
Brigitte Wilderman –
I’m happy with the training progress! The step-by-step approach really helped me understand solar water desalination. Just curious, is the slope of the glass surface critical for the process? And, how does changing the angle affect the distillation efficiency?
MR CFD Support –
Yes, the slope of the glass surface is indeed crucial for the solar water desalination process. It influences the cooling rate of the vapor and facilitates the condensation and collection of distilled water. Adjusting the angle of the glass surface impacts how efficiently the system can condense vapor into water and how gravitational forces assist in collecting the pure water. Different angles could be simulated to optimize the process for maximum efficiency.
Osbaldo Schmitt –
The module has definitely expanded my comprehension of solar desalination dynamics. It effectively illustrated the distillation process and water movement through the surface. Excellent work in clarifying such a complex concept!
MR CFD Support –
Thank you for taking the time to leave such a positive review! We are thrilled to hear that our simulation training module was able to enhance your understanding of solar water desalination. Our goal is to make complex concepts accessible and clear, and it’s great to know we succeeded with this module. If you ever have more queries or need further assistance, don’t hesitate to reach out!
Antwan Leannon DDS –
I really appreciate how the solar desalination CFD simulation handles complex processes effectively. Solar energy as a renewable source for water purification is truly impressive.
MR CFD Support –
Thank you for your kind words! We’re glad you recognize the importance of sustainable solutions in our simulations. It’s our goal to provide high-quality and environmentally-conscious CFD training products.
Claire Armstrong –
What a fantastic educational experience! It clearly illuminated the intricacies of the desalination process and how solar energy can be utilized effectively. The detailed methodology provided a strong foundation for understanding the simulation, and I was especially impressed by the use of UDF for surface evaporation which simplified the process within the Species Transport method. Thanks to MR CFD for a thorough and practical learning tool!
MR CFD Support –
Your appreciation means a lot to us, and we are thrilled that our Simulation Training on Solar Water Desalination has offered you valuable insights. Thank you for recognizing the efforts we put into simplifying complex processes like desalination for better understanding. We hope that our product continues to assist you in expanding your knowledge and expertise. If you have any further questions or need assistance, feel free to reach out!
Thea Runolfsdottir –
I just finished reviewing the Solar Water Desalination by Species Transport, 2-D ANSYS Fluent training product. It explains the solar desalination processes really well, and I grasped the concept of species transport without any issues! Super impressed with the structured mesh details and how they influenced the simulation results. Thanks, MR CFD, for creating such a detailed study!
MR CFD Support –
Thank you so much for your positive feedback! We’re delighted to hear that our training on Solar Water Desalination by Species Transport was clear and helpful for understanding the simulation process. Knowing that the details on structured mesh grabbed your attention and helped you appreciate the intricacies of the analysis is very rewarding for our team. If you have any more questions or need further assistance with your CFD learning journey, don’t hesitate to reach out. Thanks again!
Jaylan Toy IV –
I’ve been trying to understand how the UDF for surface evaporation enhances the accuracy of the simulation. Can you explain?
MR CFD Support –
Certainly! The UDF (User-Defined Function) allows for more accurate representation of surface evaporation processes by incorporating specific calculations that reflect the physical characteristics of evaporation due to solar energy. It helps in simulating the phase change from liquid water to vapor, which highly impacts the system’s performance in a real solar desalination unit.
Modesta Hauck –
The product descriptions look really detailed and thoughtful. Thank you!
MR CFD Support –
Thank you so much for your kind words! We’re thrilled to hear that you’re satisfied with the level of detail and thought put into our product descriptions. If you have any more feedback or ever need assistance, feel free to reach out.